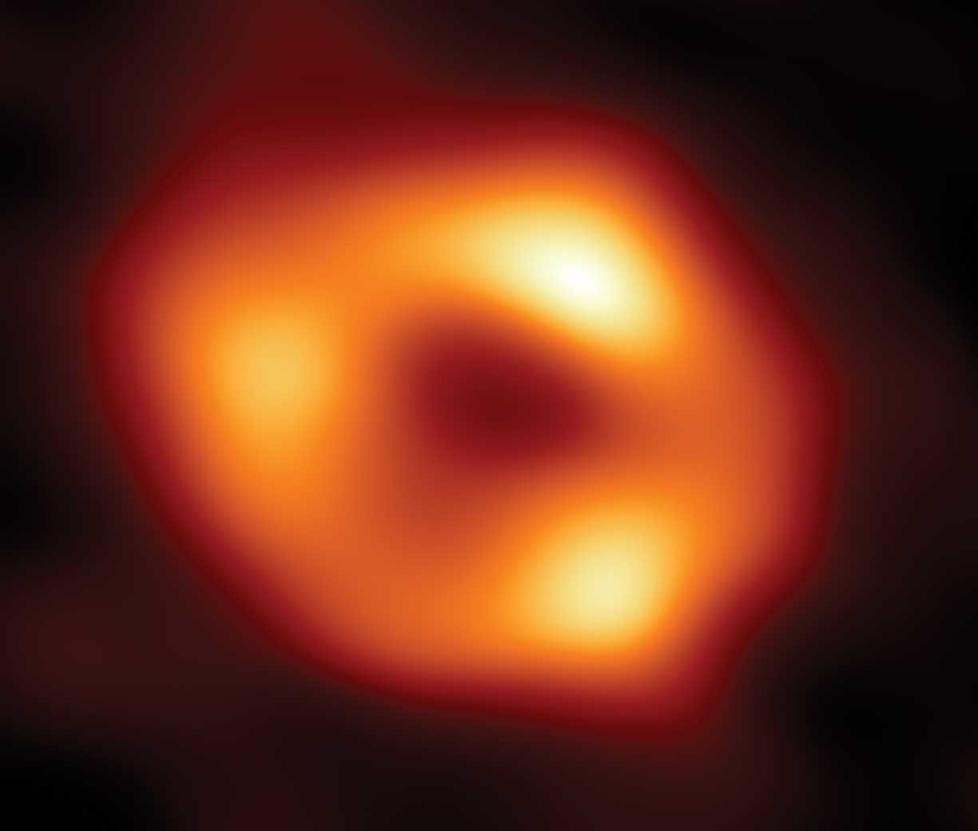
Mathematicians Tied to Princeton Prove Stability of Black Holes

The Event Horizon Telescope has created a single image of the supermassive black hole at the center of our galaxy, called Sagittarius A*.

EHT Collaboration

A group of mathematicians with strong Princeton ties has proven that black holes are stable — confirming long-held assumptions related to general relativity — through a series of papers totaling approximately 2,100 pages. 

“Stability is a fundamental issue; in a sense you are proving that these things are real. It’s a test of reality, as I like to call it,” says Sergiu Klainerman, a mathematics professor at Princeton. Jérémie Szeftel — a former postdoctoral researcher of Klainerman’s who went on to be an instructor and visiting professor at Princeton and is now a researcher at the Sorbonne — and Klainerman led the project.

Together, the works prove that even if a black hole experiences a perturbation — a deviation in motion — it will eventually converge to a similar, nearby state.

“This is an astonishing body of work,” says Eliot Quataert, a professor of astrophysical sciences at Princeton who was not involved. “If black holes had turned out not to be stable, it would have meant that probably something was wrong in our interpretation of a very, very wide range of phenomena.”

Two of the papers, which are all mathematical proofs, have been published in Annals of PDE, while a third has been peer-reviewed and is awaiting publication in Pure and Applied Mathematics Quarterly. The other two proofs, including a 900-page paper, are still under review. Elena Giorgi, a former postdoc at Princeton’s Gravity Initiative who is now a professor at Columbia, is co-author of the 900-pager with Klainerman and Szeftel; Dawei Shen, a Ph.D. student of Szeftel’s, authored the other yet-to-be-reviewed proof. 

Mathematicians have spent decades trying to do what these collaborators have now accomplished. It goes back to 1915, when Albert Einstein proposed his Theory of General Relativity, which theorizes how gravity affects the fabric of spacetime, but it wasn’t until 1963 that mathematician Roy Kerr discovered one of the solutions to the Einstein equations: rotating black holes. 

Klainerman says Kerr’s discovery gave mathematicians “a monumental task: to test the physical reality of these solutions.”

Since then, there have been incremental developments, including from Klainerman himself. (In 1993, he and collaborator Demetrios Christodoulou *71 proved the stability of Minkowski space — essentially empty space.) The mathematicians are quick to point out that these new proofs represent a culmination of work by many people over many years.

In the meantime, physicists assumed that black holes are stable, but that didn’t necessarily mean they were right. “[Physicists] really only looked at some specific perturbations … and they show[ed] that those perturbations were not exponentially growing in time,” says Giorgi. “But it doesn’t really prove that it’s stable,” as general perturbations weren’t taken into account.

She points to Minkowski space as an example of when physicists’ assumptions didn’t capture the whole picture. In that case, mathematicians “were able to find some effects that were not known before,” according to Giorgi.

“This is a nice … example of when mathematics can give back to physics,” she says. “Mathematics can actually help in understanding.”

And while the new works represent a big step forward, there is still a restriction that could be further expanded upon and developed: The solution only holds true for slowly rotating black holes of a certain type, called Kerr black holes (named after Roy Kerr). 

“Essentially, the modeling of black holes is based on Kerr,” says Klainerman. He also notes that “in most of our work, we don’t need that [slowly rotating] condition.”

Klainerman expects that the stability of black holes will be definitively proven without this restriction within five years. “I think it’s a matter of time [before we] have the whole thing.”

With a chuckle, the 72-year-old Klainerman tells PAW, “I’m getting old. I’m not sure that I can keep up with all the young people in the field, but hopefully I can still work on this. I have other plans also. We’ll see.”