Was Albert Einstein Wrong?

For the part ten years, Professor Robert Dicke ’39 and his colleagues at Princeton and elsewhere have been devising tests for the scalar-tensor theory of gravitation. The scalar-tension theory is a modification of Einstein’s theory of general relativity. In 1965, 1967, and 1970 there were several journalistic flurried about one or another of the experiments concerning the theory. This search for experimental verification has involved Dicke in investigating the shape and composition of the sun, in projects which have ended with putting instruments on the moon, and in speculations on the origins of the universe.

The scalar-tensor theory does not attempt to replace general relativity. Rather, it supplements general relativity with a second gravitational effect. In general relativity, the strength of gravitation is constant in space and time. According to scalar-tensor theory, the strength of gravitation weakens with time.

There have been a number of papers publishes on the subject of the theory since its first, dramatic appearance in an article by C. Brans and Dicke in The Physical Review in 1961. Partly, this is so because relativity is a glamorous subject. Most of the papers address themselves to formal theoretical problems concerning the statement of the scalar-tensor theory. And Dicke himself published a different form of the theory a year after the original published version.

The name scalar-tensor refers to the character of the fields which are used in the theory to describe gravitation. An example of a scalar field is air pressure in a room. Every point in the room has a definite, measurable pressure. An example of a tensor field would be something like stress in the earth’s surface, or the stretching of a piece of rubber. But even these three-dimensional analogies are misleading because both of these fields, the scalar and the tensor, exist in four-dimensional space with time as the fourth dimension.

Using a scalar to express gravitation goes back to Newton. The representation of gravitation by means of a tensor is due to Einstein. That’s the central core of relativity. The scalar-tensor theory weds Newton’s gravitational theory to Einstein’s relativistic theory. The importance of adding the scalar is that it allows the strength of gravitation locally to reflect the structure of the whole universe. In general relativity, local effects only express local structures. For most laboratory purposes the differences between the one and the other are negligible. But in cosmology, there are enormous and significant differences between the Einstein formulation and the scalar-tensor theory.

Dicke first became interested in gravitation in the late fifties when he was on sabbatical leave at Harvard. Before then his research involved precision measurements on atomic systems. During World War II he worked on the development of microwave radar, and his dissertation had been on nuclear physics. While at Harvard, Dicke concluded that little experimental work had been done on gravitation. When he returned to Princeton the following year he set up a small scale experiment called the Eötvös experiment, after the Hungarian who first performed a different version of it in 1889.

The Eötvös experiment addressed itself to the following question: given two bodies of different composition (for example, aluminum and gold) do they experience the same gravitational acceleration? Few experiments are simpler in principle, harder in practice, and so far-reaching in implication, Dicke notes. Eötvös was able to show that different composition bodies will fall with the same acceleration to an accuracy of one part in one hundred million. Dicke and his colleagues improved upon this and showed an accuracy of one part in one hundred billion. Eötvös’ formulation of the experiment showed bodies in a balance pulled by gravitation towards the earth. Dicke and his colleagues had the particles pulled towards the sun with the earth’s motion. The Princeton group examined the motions of gold and aluminum weights as they fell along with the earth towards the sun.

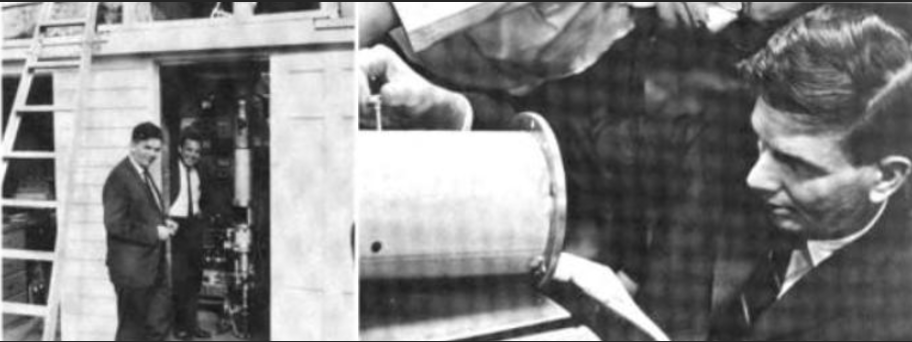
What gave the physicists the most trouble was the practical problems concerned with the experiment. A pit somewhere in what is now the Jadwin Cage parking lot was dug to house the equipment and instruments. One of the biggest difficulties was assuring a uniformity of temperature within the pit. All the equipment was monitored from above ground by remote control.

These photographs show Professor Dicke and a former colleague, Professor Mark Goldenburg, with the device used for measuring the shape of the sun. It is perhaps alarming that a physicist investigating theories of gravity took the precaution of chaining his ladder to the roof.

Princeton Alumni Weekly. April 27, 1971.

 After the Eötvös experiment was finished (it took about five years to run) Dicke turned his attention to the question of solar oblateness—the slight elliptical shape of the sun as the rotation axis is shortened by gravitational forces. With two former colleagues, he designed several experiments to measure solar oblateness. Here is another example of how theory plays a critical role in the nature of experiment. In looking for a test of the scalar-tensor theory, Dickens discovered that a small part of the orbital disturbance of mercury, previously accounted for only by relativistic effects, might be explained by solar oblateness, a distortion in the sun’s shape.

The scalar-tensor theory also has vast implications for theories of the origin and age of the universe. Once implication of the scalar-tensor theory is that the strength of the gravitational interaction decreases at a rate as great as one part in a hundred billion per year. Attempts are being made to measure these changes upon the motion of the moon. The moon ought to revolve around the earth more slowly over the hundreds of millions of years according to the scalar-tensor theory. Physicists and astronomers from Princeton and other places persuaded NASA to put corner reflectors on the moon to measure this and other effects. These corner reflectors are small quartz corners of cubes ground to optical precision. They bounce back laser beams and measure the distance from the earth to a point on the moon with an accuracy of thirty-four centimeters. Now that these corner reflectors are up there, they will last for over one hundred years and give extremely accurate observations for an experimentally significant time span. The interpretation of these highly accurate results will, however, lead back to the unresolved differences between physicists.

Dicke notes that the tidal effects upon the moon may be more complex than were originally thought. And there may be other interesting relativistic effects upon the moon’s motion. The earth-moon system can also be conceived of as a giant Eötvös balance, with the earth and the moon being bodies of different composition attracted to the sun. Under the scalar-tensor theory a slight difference in acceleration towards the sun would be expected. Another implication of the scalar-tensor theory is that the moon, and the earth, should be expanding gradually as the force of gravity weakens. There may be evidence on the moon of expansion. The rills could be expansion cracks.

The age and origin of the universe also differ considerably under scalar-tensor theory and under general relativity. Scalar-tensor theory predicts that the sun would have been hotter in the past, whereas according to general relativity and the sun should gradually warm up with time as it burns hydrogen and produces helium. The matter of the helium content of starts, like the sun, is also relevant to the scalar-tensor theory and to questions concerning the age and beginnings of the universe. The scalar-tensor theory postulates a slightly younger universe than general relativity. While it would be difficult to prove or disprove scalar-tensor theory on the basis of deductions of the age of the universe or the composition of distant stars, a positive proof of scalar-tensor theory on other grounds would provide an enormously useful key to interpretations of the cosmic fireball and the origin of the universe. Dicke is so far unimpressed by results which argue against the scalar-tensor theory. Recently, unmanned Mariner satellites passing behind the sun have sent back results which seem to support the theory of general relativity over scalar-tensor theory. According to scalar-tensor theory the bending of the Mariner radio signal by the sun’s gravitation should differ significantly from the same effect calculated under the theory of general relativity. Early results agree more closely with the predictions of general relativity. However, Dicke argues, the path of the Mariner itself was not reliable. The space craft was buffeted about by light pressure, solar wind, and gas jets. The orbit of the Mariner itself varied significantly. Dicke hopes that other experiments which bounce back radar off the planets, particularly off Venus and Mercury, will show more positive results. Dicke also thinks that the whole history of solar eclipse observations should be reviewed. The data has not been precise, he argues, and he would like to see a solar eclipse expedition sent from Princeton.

But what do theoretical physicists do? They design experiments which test their theories. They argue, persuade, and lecture on their theories. They even take wagers on the subject. Tacked on the wall of Dicke’s office in the new Jadwin physics building is a small piece of paper with three muddy signatures at the bottom. “Witnessed: that when the bending of solar light is established as being less than 0.93 + 0.03 = 0.96 with a confidence of 99% … a payment of one new ten dollar bill will be made. Signed J. Wheeler, R. H. Dicke and Witness.” Settling this bet presupposes agreement upon the observations. And then there is the trickier matter of “with a confidence of 99%.” The two principals will most likely be keeping their money in their pockets for some time yet, nature being what it is, and theoretical physicists being what they are.

This was originally published in the April 27, 1971 issue of PAW.